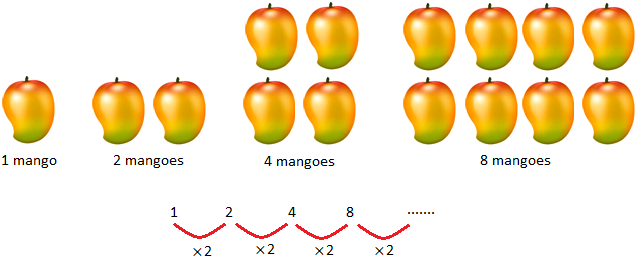
Arithmetic Sequence Phrase Issues

This lesson will present you tips on how to clear up a wide range of arithmetic sequence phrase issues.

Instance #1:

Suppose that you simply and different college students in your faculty take part in a fundraising occasion that’s attempting to lift cash for “underprivileged” kids . The college begins with $2000 in donations. Then, every scholar should elevate no less than $45 in pledges. What number of college students participated if the minimal sum of money raised is 6050?

Resolution

To unravel this drawback, we want the arithmetic sequence system proven under.

a_n = a₁ + (n – 1)d

a₁ = starting quantity  = 2000

a_n = closing quantity  = 6050

d = sum of money raised by every scholar

6050 = 2000 + (n – 1) × 45

6050 – 2000 = 2000 – 2000 + (n – 1) × 45

4050  = (n – 1) × 45

4050 = 45n – 45 

4050 + 45 = 45n – 45 + 45

4095 = 45n

n = 4095 / 45 = 91

91 college students participated within the fundraising occasion.

Instance #2:

The second time period of an arithmetic sequence is -3 and the sixth time period of the arithmetic sequence is 9. Discover the thirtieth time period of the arithmetic sequence.

Resolution

Discover the second time period

a_n = a₁ + (n – 1)d

a₂ = a₁ + (2 – 1)d

a₂ = a₁ + d

For the reason that second time period is -3,  -3 = a₁ + d

Discover the sixth time period

a_n = a₁ + (n – 1)d

a₆ = a₁ + (6 – 1)d

a₆ = a₁ + 5d

For the reason that sixth time period is 9,  9 = a₁ + 5d

We now have a system of equations to unravel

a₁ + 5d = 9 (equation 1)

a₁ + d = -3 (equation 2)

Do equation 1 minus equation 2

a₁ – a₁ + 5d – d = 9 – -3

4d = 9 + 3

4d = 12

d = 12 / 4 = 3

a₁ + d = -3

a₁ + 3 = -3

a₁ = -3 – 3

a₁ = -6

a₃₀ = a₁ + (30 – 1)3

a₃₀ = -6 + (29)3

a₃₀ = -6 + 87

a₃₀ = 81

Difficult arithmetic sequence phrase issues

Instance #3

Buses in your route run each 7 minutes from 6:30 A.M. to 10:00 A.M. You get to the bus cease at 7:56 A.M. How lengthy will you need to look ahead to a bus?

Resolution

First, you’ll want to discover out primarily based on the time you arrived which bus you missed (if any) or which bus is coming for those who missed the final one.

a_n = a₁ + (n – 1)d

a₁ = first bus at 6:30 = 0 minute ready time

a_n = ready time for the nth bus you want, which can be equal to the time you arrive on the station.

a_n = 7.56 A.M. – 6:30 A.M. = 86 minutes

d = ready time earlier than the subsequent bus

n = the bus that you’ll want to look ahead to = ?

86 = 0 + (n – 1)7

86 = 7n – 7

86 + 7 = 7n – 7 + 7

93 = 7n

n = 93 / 3 = 13.285

Discover that 13 < 13.285 < 14

Which means that you arrived after the thirteenth bus had left and earlier than the 14th bus made it to the bus cease. You need to then look ahead to the 14th bus!

Now, discover out what time the 14th bus is coming.

a₁₄ = 0 + (14 – 1)7

a₁₄ = (13)7 = 91 minutes

You arrived 86 minutes after the primary bus and the subsequent bus is coming 91 minutes after the primary bus. Due to this fact, you have to wait 91 – 86 minutes or 5 minutes.

Instance #4

The arithmetic imply of two phrases in an arithmetic sequence is 56.

1) Discover the opposite time period if one time period is 72

2) Discover the a centesimal time period if the primary time period is 8.

Resolution

Suppose 9, 15, 21, 27, … is an arithmetic sequence.

Discover that (9 + 21) / 2 = 30 / 2 = 15 and (15 + 27) / 2 = 42 / 2 = 21

15 or the time period between 9 and 21 is the arithmetic imply of 9 and 21

21 or the time period between 15 and 27 is the arithmetic imply of 15 and 27

Typically, if a₁, a₂, a₃, a₄, a₅, … is an arithmetic sequence, a₂ for instance, is the arithmetic imply of a₁ and a₃

Now, you’re prepared to unravel the issue. 

(a₁ + a₃) / 2 = 56

Multiply either side by 2

a₁ + a₃ = 112

For the reason that different time period is 72, we get 72 + a₃ = 112

a₃ = 112 – 72 = 40

Due to this fact, the sequence has these phrases: 8, … , 40, 56, 72, …

a_n = a₁ + (n – 1)d

a₁ = 8

n = 100

d = 16 since 56 – 40 = 16 and 72 – 56  = 16

a₁₀₀ = ?

a₁₀₀ = 8 + (100 – 1)16

a₁₀₀ = 8 + (99)16

a₁₀₀ = 8 + 1584

a₁₀₀ = 1592