Rational Numbers – Definition and Examples

Rational numbers are any numbers that may be written as a fraction. In different phrases, you possibly can rewrite the quantity so it would have a numerator and a denominator.

Examples of rational numbers

Now, why are 2 and 0 rational numbers as properly?

It’s as a result of 2 and 0 might be written as

x might be any quantity since 0 divided by any quantity is zero. Discover although that in keeping with the definition x can’t be zero!

Decimal growth of rational numbers

We are able to additionally write rational numbers as decimals.

We do that by performing a fast division. We divide the numerator by the denominator.

As an example,

Dividing by 100 or some other energy of 10 is an easy course of.

In case you are dividing by 10, simply transfer the decimal level one place to the left.

In case you are dividing by 100, simply transfer the decimal level 2 locations to the left.

and so forth…

Shifting that two locations to the left deliver the decimal level proper earlier than the 4.

A rational quantity can have both repeating decimal growth or terminating decimal growth.

Repeating decimal growth:

A decimal growth during which the numbers repeat in precisely the identical order.

For instance, 0.251251251251 is a repeating decimal growth as a result of 251 retains repeating in the identical order.

Terminating decimal growth:

A decimal growth that ends in all zeros.

For instance, 0.150 is a terminating decimal growth.

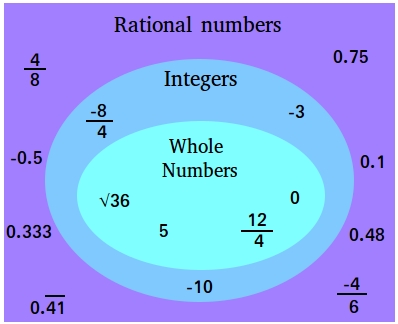
Here’s a determine summarizing rational numbers or the “various kinds of rational numbers”

• Pure numbers are rational numbers since any pure quantity might be written within the kind a/b. For instance, 2 might be written as 2/1.

• Complete numbers are rational numbers since any entire quantity might be written within the kind a/b. For instance, 9 might be written as 9/1.

• Integers are rational numbers since any integer might be written within the kind a/b. For instance, -8 might be written as -8/1.

• Non-terminating decimal numbers with repeating patterns are rational numbers. For instance, 0.2121212121 is a rational quantity since it may be written as 7/33.

• Terminating decimal numbers are rational numbers. For instance, 0.75 is a rational quantity since it may be written as 3/4.

• Easy fractions whose numerators and denominators are entire numbers are rational numbers. For instance, the fraction 2/5 is a rational quantity. Nevertheless, the fraction 1.3/5 is just not a rational quantity since 1.3 is just not an integer in keeping with the definition of a rational quantity. 

Rational numbers FAQs

Are rational numbers integers?

No. 0.6666666 is a rational quantity. Nevertheless, it’s not an integer. Some rational numbers are integers. For instance, -8/1, 5/1 are rational numbers and integers as properly since -8/1 = -8 and 5/1 = 5. Nonetheless, each integer is a rational quantity!

Are rational numbers actual numbers?

Sure, rational numbers are actual numbers since actual numbers embody all units of numbers besides complicated numbers.

Are rational numbers unfavourable?

No, not essentially!There are unfavourable rational numbers and optimistic rational numbers resembling -1/2, 15/6, -4/3, 6/20.

Is 3.14 a rational quantity?

3.14 is a well-known irrational quantity generally known as pi. 3.14 has an infinite variety of digits after the decimal level that would not have repeating patterns. Subsequently, pi can’t be written as a ratio or be a rational quantity.

How are you aware if a quantity is just not rational?

As a rule of thumb, if the quantity has a bunch of various digits after the decimal level with no particular sample, the quantity is just not rational.

Check your understanding of this lesson with the rational numbers quiz under.